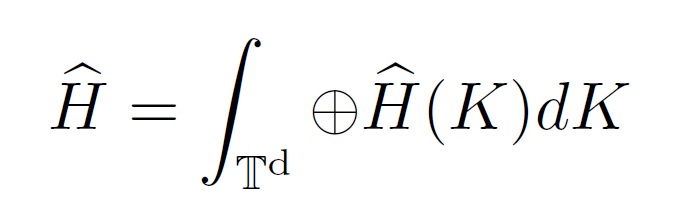NANOSYSTEMS: PHYSICS, CHEMISTRY, MATHEMATICS, 2019, 10 (5), P. 511–519
Analytic description of the essential spectrum of a family of 3 × 3 operator matrices
T. H. Rasulov – Faculty of Physics and Mathematics, Bukhara State University M. Ikbol str. 11, 200100 Bukhara, Uzbekistan; rth@mail.ru
N. A. Tosheva – Faculty of Physics and Mathematics, Bukhara State University M. Ikbol str. 11, 200100 Bukhara, Uzbekistan; nargiza_n@mail.ru
We consider the family of 3 × 3 operator matrices H(K), K ∈ Td := (-π; π)d arising in the spectral analysis of the energy operator of the spin-boson model of radioactive decay with two bosons on the torus Td. We obtain an analog of the Faddeev equation for the eigenfunctions of H(K). An analytic description of the essential spectrum of H(K) is established. Further, it is shown that the essential spectrum of H(K) consists the union of at most three bounded closed intervals.
Keywords: family of operator matrices, generalized Friedrichs model, bosonic Fock space, annihilation and creation operators, channel operator,
decomposable operator, fiber operators, the Faddeev equation, essential spectrum, Weyl criterion.
DOI 10.17586/2220-8054-2019-10-5-511-519