Nanosystems: Phys. Chem. Math., 2022, 13 (1), 45–49
Two-dimensional non-topological solutions of Maxwell’s equations in a medium of strained carbon nanotubes with impurities
Mikhail B. Belonenko – Volgograd State University, Volgograd, Russia; belonenko@volsu.ru
Natalia N. Konobeeva – Volgograd State University, Volgograd, Russia; byana_nn@volsu.ru
Corresponding author: Mikhail B. Belonenko, belonenko@volsu.ru
DOI 10.17586/2220-8054-2022-13-1-45-49
PACS 42.65.Tg, 61.72.-y, 73.63.Fg
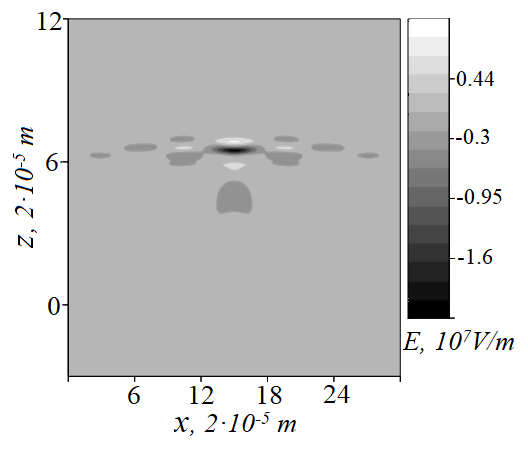
ABSTRACT In this work, we investigate the few-cycle optical pulses with Gauss and Bessel profile in strained carbon nanotubes with impurities. We consider a multi-level impurity in which the energy levels are well separated from the conduction and valence bands of carbon nanotubes. The effect of the impurity parameters on the electromagnetic pulse is analyzed. Also, we investigate the influence of the value of the mechanical stretching on the few-cycle pulse shape.
KEYWORDS carbon nanotubes, impurities, mechanical tension, few-cycle pulse
ACKNOWLEDGEMENTS Authors thank the Ministry of Science and Higher Education of the Russian Federation for the numerical modeling support under the government task (0633-2020-0003).
FOR CITATION Belonenko M.B., Konobeeva N.N. Two-dimensional non-topological solutions of Maxwell’s equations in a medium of strained carbon nanotubes with impurities. Nanosystems: Phys. Chem. Math., 2022, 13 (1), 45–49.
[In Russian] М.Б. Белоненко, Н.Н. Конобеева
Двумерные нетопологические решения уравнений Максвелла в среде нагруженной углеродными нанотрубками с примесями
АННОТАЦИЯ Исследуется нелокальная краевая задача для системы обыкновенных интегро-дифференциальных уравнений с импульсными эффектами, вырожденным ядром и максимумами. Краевая задача задается интегральным условием. Используется метод последовательных приближений в сочетании с методом сжимающего отображения. Доказаны существование и единственность решения краевой задачи. Показана непрерывная зависимость решения от правой части краевого условия.
КЛЮЧЕВЫЕ СЛОВА импульсные интегро-дифференциальные уравнения, нелокальное условие, последовательные приближения, существование и единственность, непрерывная зависимость решения.