Nanosystems: Phys. Chem. Math., 2024, 15 (3), 315–324
Energy and spectral radius of Zagreb matrix of graph with applications
Shashwath S. Shetty – Manipal Institute of Technology, Manipal Academy of Higher Education, Manipal, Karnataka, India; shashwathsshetty01334@gmail.com
K. Arathi Bhat – Manipal Institute of Technology, Manipal Academy of Higher Education, Manipal, Karnataka, India; arathi.bhat@manipal.edu
Corresponding author: K. Arathi Bhat, arathi.bhat@manipal.edu
DOI 10.17586/2220-8054-2024-15-3-315-324
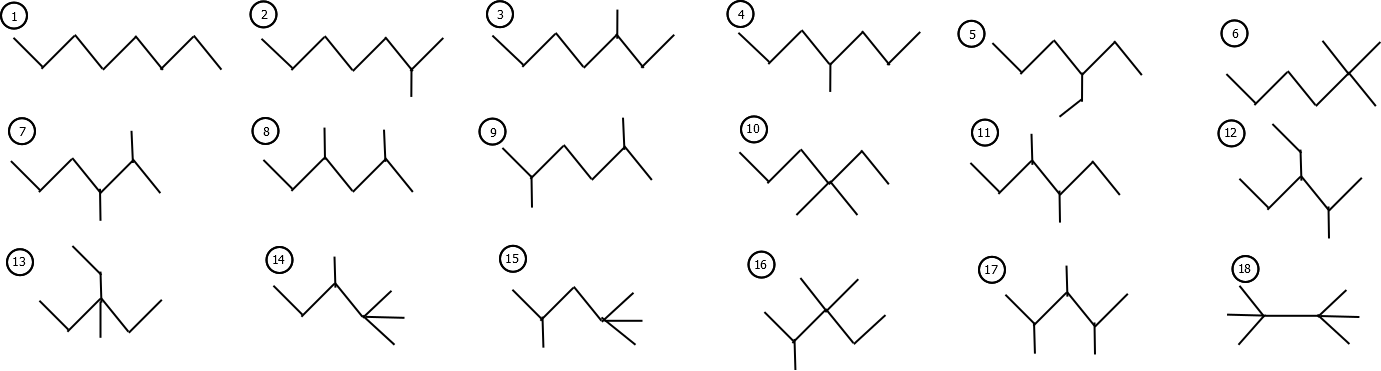
ABSTRACT The Z-matrix of a simple graph Γ is a square symmetric matrix, whose rows and columns correspond to the vertices of the graph and the ijth entry is equal to the sum of the degrees of ith and jth vertex, if the corresponding vertices are adjacent in Γ, and zero otherwise. The Zagreb eigenvalues of Γ are the eigenvalues of its Z-matrix and the Zagreb energy of Γ is the sum of absolute values of its Zagreb eigenvalues. We study the change in Zagreb energy of a graph when the edges of the graph are deleted or rotated. Suppose Γ is a graph obtained by identifying u ∈ ν(Γ1) and v ∈ ν(Γ2) or adding an edge between u and v, then it is important to study the relation between Zagreb energies of Γ1, Γ2 and Γ. The highlight of the paper is that, the acentric factor of n-alkanes appear to have a strong positive correlation (where the correlation coefficient is 0.9989) with energy of the Z-matrix. Also, the novel correlation of the density and refractive index of n-alkanes with spectral radius of the Z-matrix has been observed.
KEYWORDS spectral radius, energy, Zagreb matrix, acentric factor, density, refractive index
ACKNOWLEDGEMENTS We acknowledge the Institution for overall support. The authors sincerely appreciate the reviewers for all the valuable comments and suggestions, which helped to improve the quality of the manuscript.
FOR CITATION Shashwath S. Shetty, K. Arathi Bhat Energy and spectral radius of Zagreb matrix of graph with applications. Nanosystems: Phys. Chem. Math., 2024, 15 (3), 315–324.
[In Russian] Шашват С. Шетти, К Арати Бхат
Энергия и спектральный радиус Загребской матрицы графа с приложениями
АННОТАЦИЯ Z-матрица простого графа Γ представляет собой квадратную симметричную матрицу, строки и столбцы которой соответствуют вершинам графа, а ij-й элемент равен сумме степеней i-й и j-й вершины, если соответствующие вершины смежны. в Γ и нулю в противном случае. Загребские собственные значения Γ являются собственными значениями его Z-матрицы, а загребская энергия Γ представляет собой сумму абсолютных значений его загребских собственных значений. Мы изучаем изменение загребской энергии графа при удалении или повороте ребер графа. Предположим, что Γ – граф, полученный отождествлением u ∈ ν(Γ1) и v ∈ ν(Γ2) или добавлением ребра между u и v, тогда важно изучить связь между загребскими энергиями Γ1, Γ2 и Γ. Изюминкой статьи является то, что ацентрический фактор н-алканов, по-видимому, имеет сильную положительную корреляцию (где коэффициент корреляции составляет 0,9989) с энергией Z-матрицы. Также наблюдалась новая корреляция плотности и показателя преломления n-алканов со спектральным радиусом Z-матрицы.
КЛЮЧЕВЫЕ СЛОВА спектральный радиус, энергия, загребская матрица, ацентрический фактор, плотность, показатель преломления