NANOSYSTEMS: PHYSICS, CHEMISTRY, MATHEMATICS, 2018, 9 (2), P. 225–243
Cyclic-periodic ZRP structures. Scattering problem for generalized Bloch functions and conductivity
S. Leble – Immanuel Kant BFU, Al. Nevsky st.14, Kaliningrad, 236016, Russia; lebleu@mail.ru
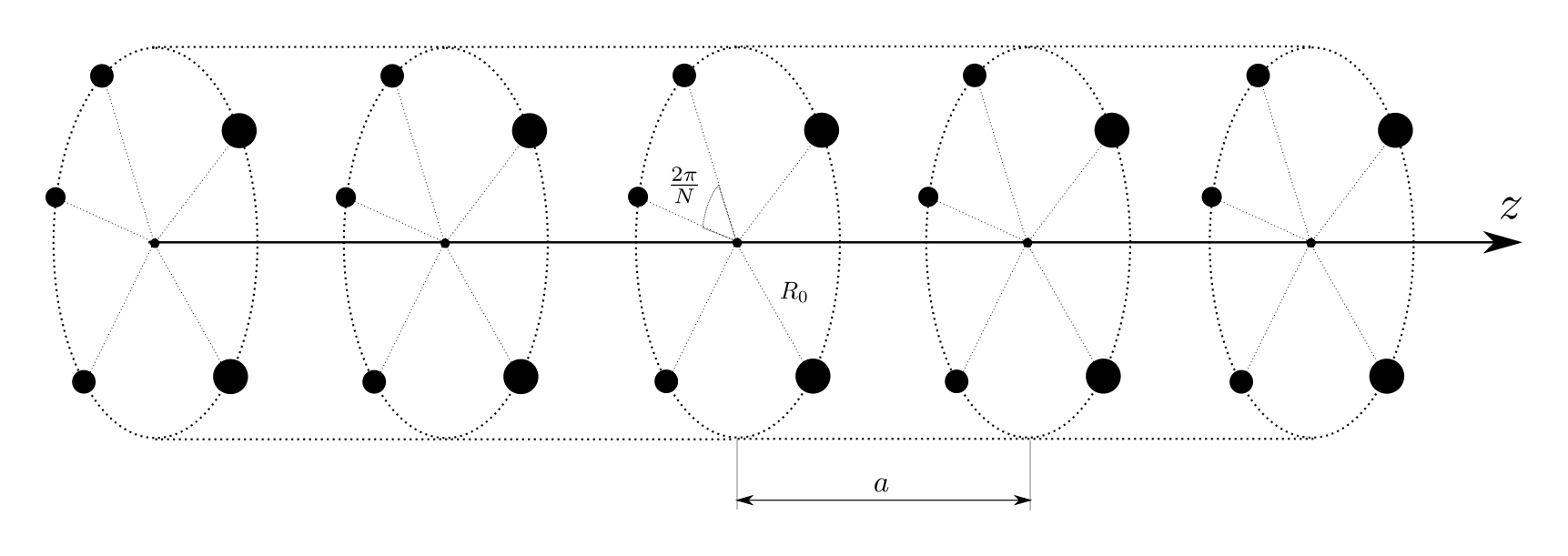
Problems of quantum description of nanostructures transport properties is investigated in a framework of the structure symmetry group. Corresponding states of conductivity electrons are defined as irreducible representations of the group. The right/left Bloch functions are written and the Floquet theorem is formulated. The results are used for formulating the zero range potential (ZRP) conditions for arbitrary orbital quantum number whose construction is presented via Darboux dressing chain built in a space of distributions. The electron spin variables are taken into account. A spectrum of the non-relativistic Hamiltonian with a system of a nanostructure ZRPs is found from the matrix eigenvalue problem. The scattering problem on an extra ZRP is formulated in terms of the right/left Bloch functions. As an example, the discrete spectrum and scattering on N-ZRP-centers is solved and compared with experimental data for benzene molecule.
Keywords: Nanostructures, zero-range potential, Bloch wave, scattering problem, charge transport.
PACS 03.65.Nk, 05.60.-k
DOI 10.17586/2220-8054-2018-9-2-225-243