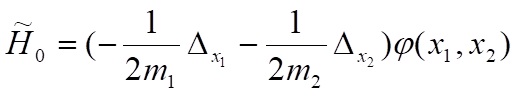Nanosystems: Phys. Chem. Math., 2023, 14 (5), 505–510
On the spectrum of the two-particle Schrödinger operator with point potential: one dimensional case
Utkir N. Kuljanov – Samarkand State University, Samarkand; Samarkand branch of Tashkent State University of Economics, Samarkand, Uzbekistan; uquljonov@bk.ru
DOI 10.17586/2220-8054-2023-14-5-505-510
ABSTRACT In the paper, a one-dimensional two-particle quantum system interacted by two identical point interactions is considered. The corresponding Schrödinger operator (energy operator) hε depending on ε is constructed as a self-adjoint extension of the symmetric Laplace operator. The main results of the work are based on the study of the operator hε. First, the essential spectrum is described. The existence of unique negative eigenvalue of the Schrödinger operator is proved. Further, this eigenvalue and the corresponding eigenfunction are found.
KEYWORDS two-particle quantum system, symmetric Laplace operator, eigenvalue, eigenfunction, energy operator
ACKNOWLEDGEMENTS Author partially supported by [grant number FZ-20200929224] of Fundamental Science Foundation of Uzbekistan.
FOR CITATION Kuljanov U.N. On the spectrum of the two-particle Schrödinger operator with point potential: one dimensional case. Nanosystems: Phys. Chem. Math., 2023, 14 (5), 505–510.
[In Russian] У.Н. Кулжанов
О спектре двухчастичного оператора Шредингера с точечным потенциалом в одномерном случае
АННОТАЦИЯ В работе рассматривается точечно-взаимодействующая одномерная двухчастичная квантовая система. Соответствующий оператор Шредингера (оператор энергии) hε, зависящего от параметра расширения строится как самосопряженное расширение ε симметрического оператора Лапласа. Основные результаты работы основываются на изучение спектра оператора hε. Описан существенный спектр и доказано существование одного отрицательного собственного значения оператора Шредингера при положительных значениях параметра расширения. Более того, найдены отрицательные собственные значение и соответствующая собственная функция.
КЛЮЧЕВЫЕ СЛОВА двухчастичная квантовая система, симметричный оператор Лапласа, собственное значение, собственная функция, оператор энергии.