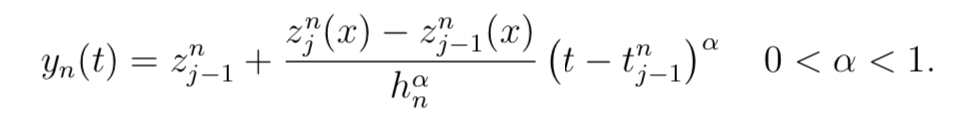Nanosystems: Phys. Chem. Math., 2024, 15 (1), 5–15
Existence and uniqueness theorem for a weak solution of fractional parabolic problem by the Rothe method
Y. Bekakra – ICOSI Laboratory, Abbes Laghrour University, Khenchela, 04000, Algeria; ayoucef.bekakra@univ-khenchela.dz
A. Bouziani – ICOSI Laboratory, Abbes Laghrour University, Khenchela, 04000; L’arbi Ben M’hidi University, Oum El Bouagui, 04000, Algeria; baefbouziani1963@gmail.com
Corresponding author: Y. Bekakra, youcef.bekakra@univ-khenchela.dz
PACS 02.60−x, 47.11.Bc, 02.30.Jr
DOI 10.17586/2220-8054-2024-15-1-5-15
ABSTRACT This paper aims to study the existence and uniqueness of a weak solution for the boundary value problem of a time fractional equation involving the Caputo fractional derivative with an integral operator. By utilizing the discretization method, we first derive some a priori estimates for the approximate solutions at the points (x, tj). We then evaluate the accuracy of the proposed method to demonstrate that the implemented sequence of α-Rothe functions converges in a certain sense, and its limit is the solution (in a weak sense) of our problem. It must be pointed out that the constructed L1 scheme is designed to approximate the Caputo fractional derivative mentioned in the problem.
KEYWORDS weak solution, a priori estimates, Fractional diffusion equation, Rothe’s method
ACKNOWLEDGEMENTS The authors would like to thank the reviewer for his valuable comments and suggestions.
FOR CITATION Bekakra Y., Bouziani A. Existence and uniqueness theorem for a weak solution of fractional parabolic problem by the Rothe method. Nanosystems: Phys. Chem. Math., 2024, 15 (1), 5–15.
[In Russian] Ю. Бекакра, А. Бузиани
Теорема существования и единственности слабого решения дробной параболической задачи методом Роте
АННОТАЦИЯ Целью данной работы является исследование существования и единственности слабого решения краевой задачи дробного уравнения по времени, включающего дробную производную Капуто с интегральным оператором. Используя метод дискретизации, мы сначала получаем некоторые априорные оценки приближенных решений в точках (x, tj). Затем мы оценим точность предложенного метода, чтобы продемонстрировать, что реализованная последовательность α-функций Роте сходится в определенном смысле, а ее предел является решением (в слабом смысле) нашей задачи. Следует отметить, что построенная схема L1 предназначена для аппроксимации упомянутой в задаче дробной производной Капуто.
КЛЮЧЕВЫЕ СЛОВА слабое решение, априорные оценки, дробное уравнениедиффузии, метод Роте.