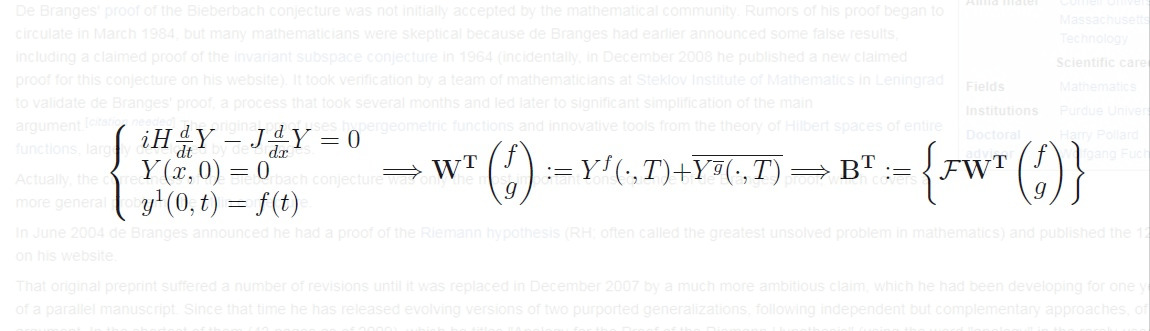NANOSYSTEMS: PHYSICS, CHEMISTRY, MATHEMATICS, 2018, 9 (2), P. 215–224
Inverse dynamic problems for canonical systems and de Branges spaces
A. S. Mikhaylov – St. Petersburg Department of V. A. Steklov Institute of Mathematics of the Russian Academy of Sciences, 7 Fontanka, St. Petersburg, 191023; St. Petersburg State University, 7/9 Universitetskaya nab., St. Petersburg, 199034 Russia; mikhaylov@pdmi.ras.ru
V. S. Mikhaylov – St. Petersburg Department of V. A. Steklov Institute of Mathematics of the Russian Academy of Sciences, 7 Fontanka, St. Petersburg, 191023; St. Petersburg State University, 7/9 Universitetskaya nab., St. Petersburg, 199034 Russia; vsmikhaylov@pdmi.ras.ru
We show the equivalence of inverse problems for different dynamical systems and corresponding canonical systems. For canonical system with general Hamiltonian we outline the strategy of studying the dynamic inverse problem and procedure of construction of corresponding de Branges space.
Keywords: inverse problem, Boundary Control method, de Branges spaces, Schrödinger operator, Dirac system, Jacobi matrices, canonical systems.
DOI 10.17586/2220-8054-2018-9-2-215-224